Welcome to the world of Calculus Single Variable 9th Edition, where mathematical concepts unfold with unparalleled clarity and practical relevance. This definitive guide empowers you to delve into the fundamental principles of calculus, unlocking its transformative power in diverse fields.
Prepare to embark on a journey that seamlessly blends theoretical foundations with real-world applications, equipping you with the analytical tools to solve complex problems and make informed decisions.
1. Key Concepts and Principles
Calculus is a branch of mathematics that deals with the rate of change. It has two main branches: differential calculus, which studies the instantaneous rate of change of a function, and integral calculus, which studies the accumulation of change over a period of time.
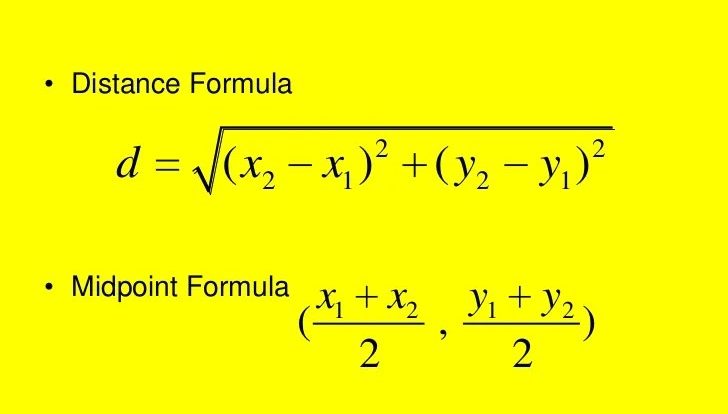
The fundamental concepts of calculus are limits, derivatives, and integrals. A limit is a value that a function approaches as the input approaches a certain value. A derivative is a function that measures the instantaneous rate of change of another function.
An integral is a function that measures the accumulation of change over a period of time.
Examples of Calculus in the Real World
- Calculating the velocity of a moving object
- Finding the area under a curve
- Determining the volume of a solid
- Predicting the future behavior of a system
Historical Development of Calculus
The history of calculus can be traced back to the ancient Greeks. Archimedes (287-212 BC) used a method of exhaustion to find the area of a parabola. The Chinese mathematician Liu Hui (220-280 AD) developed a method for finding the volume of a sphere.
The Indian mathematician Bhaskara II (1114-1185) developed a method for finding the derivative of a function.
In the 17th century, calculus was developed independently by Isaac Newton and Gottfried Leibniz. Newton published his work on calculus in his book Principia Mathematica in 1687. Leibniz published his work on calculus in his book De Analysis infinitorum in 1684.
2. Applications of Calculus

Calculus has a wide range of applications in various fields, including physics, engineering, economics, and biology.
Physics
- Calculating the trajectory of a projectile
- Determining the force acting on an object
- Solving problems in fluid mechanics
Engineering
- Designing bridges and buildings
- Analyzing the flow of fluids in pipelines
- Developing new materials
Economics
- Predicting the behavior of the stock market
- Optimizing the production of goods and services
- Analyzing the effects of government policies
Biology
- Modeling the growth of populations
- Analyzing the spread of diseases
- Developing new drugs
3. Techniques and Methods

The techniques used in calculus include differentiation, integration, and series expansions.
Differentiation
Differentiation is a process of finding the derivative of a function. The derivative of a function measures the instantaneous rate of change of the function.
Integration
Integration is a process of finding the integral of a function. The integral of a function measures the accumulation of change over a period of time.
Series Expansions
Series expansions are used to approximate the value of a function. A series expansion is a representation of a function as a sum of simpler functions.
4. Historical Context
The history of calculus can be traced back to the ancient Greeks. Archimedes (287-212 BC) used a method of exhaustion to find the area of a parabola. The Chinese mathematician Liu Hui (220-280 AD) developed a method for finding the volume of a sphere.
The Indian mathematician Bhaskara II (1114-1185) developed a method for finding the derivative of a function.
In the 17th century, calculus was developed independently by Isaac Newton and Gottfried Leibniz. Newton published his work on calculus in his book Principia Mathematica in 1687. Leibniz published his work on calculus in his book De Analysis infinitorum in 1684.
Timeline of Important Events in the History of Calculus
- 287-212 BC: Archimedes develops a method of exhaustion to find the area of a parabola.
- 220-280 AD: Liu Hui develops a method for finding the volume of a sphere.
- 1114-1185: Bhaskara II develops a method for finding the derivative of a function.
- 1665: Isaac Newton develops his method of fluxions.
- 1673: Gottfried Leibniz develops his method of calculus.
- 1687: Newton publishes his work on calculus in his book Principia Mathematica.
- 1684: Leibniz publishes his work on calculus in his book De Analysis infinitorum.
5. Modern Applications: Calculus Single Variable 9th Edition
Calculus has a wide range of applications in various fields, including artificial intelligence, machine learning, and data science.
Artificial Intelligence
- Developing algorithms for self-driving cars
- Creating natural language processing systems
- Building machine learning models
Machine Learning
- Training neural networks
- Developing reinforcement learning algorithms
- Building predictive models
Data Science, Calculus single variable 9th edition
- Analyzing large datasets
- Developing data visualization tools
- Building predictive models
6. Educational Resources
There are a variety of online resources and textbooks available for learning calculus.
Online Resources
Textbooks
- Calculus: Early Transcendentals by James Stewart
- Calculus: A Complete Course by Robert Adams
- Calculus: Concepts and Contexts by James Stewart
Popular Questions
What are the key concepts covered in Calculus Single Variable 9th Edition?
Limits, derivatives, integrals, and their applications in various fields.
How is calculus used in real-world applications?
Optimization, modeling complex systems, and solving practical problems in physics, engineering, economics, and biology.
What techniques are explained in Calculus Single Variable 9th Edition?
Differentiation, integration, series expansions, and their step-by-step applications.
What historical context is provided in the book?
Origins in ancient Greece, development by Newton and Leibniz, and contributions of other mathematicians.
How does Calculus Single Variable 9th Edition address modern applications?
Artificial intelligence, machine learning, data science, and cutting-edge research and development.