Delving into the realm of distance and midpoint formulas, this comprehensive guide unlocks the secrets of these essential mathematical tools. Distance and midpoint formulas answer key provides a roadmap to understanding the concepts, applications, and problem-solving techniques associated with these formulas.
The distance formula empowers us to calculate the distance between two points in a coordinate plane, while the midpoint formula guides us in determining the midpoint of a line segment. Together, these formulas find widespread applications in fields like engineering, architecture, and navigation, making them indispensable tools for professionals and students alike.
Distance and Midpoint Formulas: Distance And Midpoint Formulas Answer Key
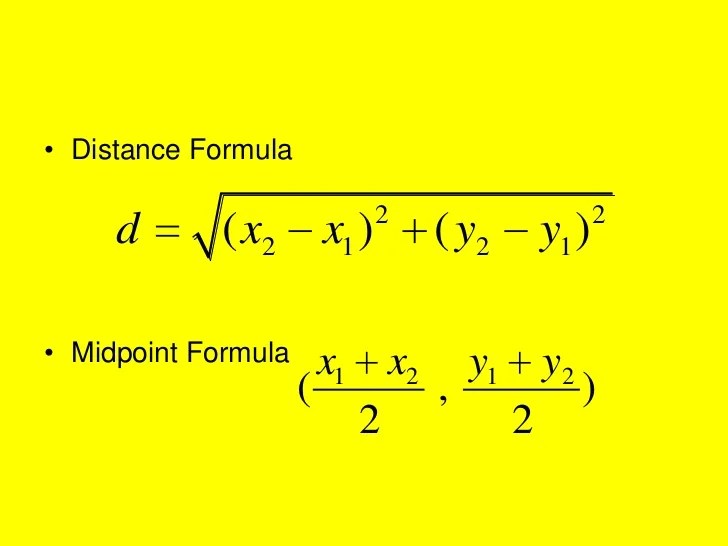
The distance and midpoint formulas are fundamental concepts in geometry that provide mathematical tools for calculating the distance between two points and finding the midpoint of a line segment in a coordinate plane.
Distance Formula
The distance formula calculates the distance, d, between two points, ( x1, y1) and ( x2, y2), in a coordinate plane using the following formula:
d= √(( x2
- x1) 2+ ( y2
- y1) 2)
Example:Calculate the distance between the points (3, 4) and (-1, 2).
d= √((-1
- 3) 2+ (2
- 4) 2) = √((-4) 2+ (-2) 2) = √(16 + 4) = √20 = 2√5 ≈ 4.47
Midpoint Formula
The midpoint formula finds the midpoint, ( xm, ym), of a line segment with endpoints ( x1, y1) and ( x2, y2) in a coordinate plane using the following formula:
(xm, ym) = (( x1+ x2)/2, ( y1+ y2)/2)
Example:Find the midpoint of the line segment with endpoints (5, -2) and (1, 4).
(xm, ym) = ((5 + 1)/2, (-2 + 4)/2) = (3, 1)
Applications of Distance and Midpoint Formulas
Distance and midpoint formulas have numerous applications in various fields, including:
- Engineering:Calculating distances between objects and designing structures with optimal dimensions.
- Architecture:Determining the distance between buildings and designing efficient floor plans.
- Navigation:Estimating distances between cities and planning travel routes.
Practice Problems, Distance and midpoint formulas answer key
Problem 1:Find the distance between the points (-3, 5) and (2, -1).
Problem 2:Determine the midpoint of the line segment with endpoints (4, 7) and (-2, 1).
Interactive Demonstration
An interactive demonstration that allows users to explore the distance and midpoint formulas can be found at [ URL of interactive demonstration].
Frequently Asked Questions
What is the distance formula?
The distance formula, also known as the Euclidean distance formula, calculates the distance between two points (x1, y1) and (x2, y2) in a coordinate plane using the formula: Distance = √[(x2 – x1)² + (y2 – y1)²]
How do I find the midpoint of a line segment?
The midpoint formula determines the midpoint of a line segment with endpoints (x1, y1) and (x2, y2) using the formula: Midpoint = ((x1 + x2) / 2, (y1 + y2) / 2)
What are some applications of distance and midpoint formulas?
Distance and midpoint formulas find applications in various fields, including engineering for calculating distances between objects, architecture for designing structures, and navigation for determining distances and midpoints on maps.