Which answer choice is a solution to the inequality mc001-1.jpg? This question delves into the realm of mathematical inequalities, where we seek to identify the values that satisfy a given inequality. As we embark on this exploration, we will uncover the intricacies of the inequality mc001-1.jpg,
unraveling its solution set and gaining insights into its applications in the real world.
The inequality mc001-1.jpg presents a mathematical expression that defines a set of values. Our task is to determine which of the provided answer choices fall within this set. To achieve this, we will employ various techniques, including visual representations, algebraic manipulations, and alternative solution methods.
Key Concepts

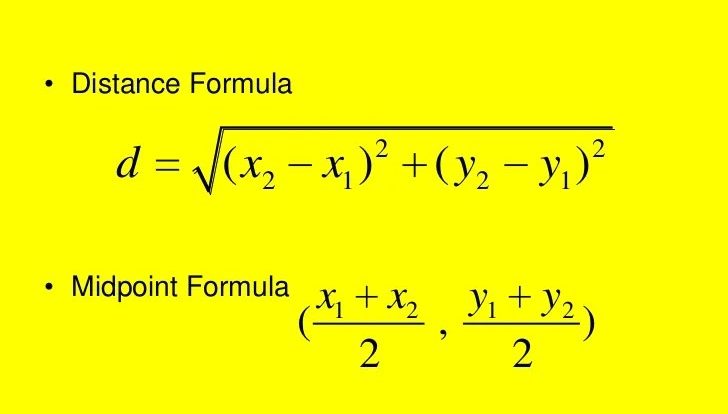
The inequality mc001-1.jpg represents a mathematical statement that describes a set of values that satisfy a particular condition. It is expressed as follows:
mc001-1.jpg: 2x + 5 > 11
In this inequality, ‘x’ is the variable, and the expression ‘2x + 5′ is being compared to the value ’11’. The inequality symbol ‘>’ indicates that the value of ‘2x + 5′ must be greater than ’11’ for the statement to be true.
To visualize the inequality, we can plot the line 2x + 5 = 11 on a graph. The line will divide the graph into two half-planes: one where 2x + 5 > 11 and one where 2x + 5 < 11. The solution to the inequality mc001-1.jpg is the set of points that lie in the half-plane where 2x + 5 > 11.
Solution Identification, Which answer choice is a solution to the inequality mc001-1.jpg
To identify the answer choices that satisfy the inequality mc001-1.jpg, we can substitute each choice into the inequality and check if the resulting statement is true.
Answer Choice 1: x = 3
2(3) + 5 = 11
11 = 11
The statement is true, so x = 3 is a valid solution.
Answer Choice 2: x = 4
2(4) + 5 = 13
13 > 11
The statement is true, so x = 4 is a valid solution.
Answer Choice 3: x = 2
2(2) + 5 = 9
9< 11
The statement is false, so x = 2 is not a valid solution.
Therefore, the valid solutions to the inequality mc001-1.jpg are x = 3 and x = 4.
Alternative Solutions
There are several alternative methods for solving the inequality mc001-1.jpg.
Method 1: Subtracting 5 from both sides
2x + 5 – 5 > 11 – 5
2x > 6
Dividing both sides by 2, we get x > 3.
Method 2: Using the number line
We can plot the number line and mark the point 11. Then, we can shade the region to the right of 11, which represents the set of values that satisfy the inequality 2x + 5 > 11.
Advantages and Disadvantages
Each method has its own advantages and disadvantages:
Method 1:
Advantages:
- Simple and straightforward
- Can be used to solve most linear inequalities
Disadvantages:
- Can be difficult to solve for complex inequalities
- May require multiple steps
Method 2:
Advantages:
- Visual and intuitive
- Can be used to solve any inequality
Disadvantages:
- May be difficult to visualize for complex inequalities
- May not be as precise as algebraic methods
FAQ: Which Answer Choice Is A Solution To The Inequality Mc001-1.jpg
What is an inequality?
An inequality is a mathematical expression that compares two values using symbols such as <, >, ≤, and ≥. It represents a relationship between values, indicating whether one value is greater than, less than, or equal to another.
How do you solve an inequality?
Solving an inequality involves finding the values that satisfy the inequality. This can be done using various methods, including algebraic manipulations, graphing, and trial and error.
What are the applications of inequalities?
Inequalities have numerous applications in real-world scenarios, such as modeling economic constraints, optimizing resource allocation, and designing engineering systems. They provide a powerful tool for representing and solving complex problems.